
They crop up in nature with surprising frequency-as in the number of petals in a flower. The Fibonacci numbers and the golden ratio often appear in mathematics-in the geometry of pentagons, for example. The golden ratio yields the golden rectangle, which can be of any size so long as the ratio of the sides is 1.618. Divide a line into two parts in such a way that the longer portion (a) divided by the smaller one (b) equals the sum of (a) + (b) divided by (a). The higher the Fibonacci numbers, the closer they are to the golden ratio of 1.618.Īnd there’s more. Now it happens that the ratios of Fibonacci numbers (2/1, 3/2, 5/3, and so on) approach the golden ratio. That comes out to about 1.618, and the decimal places continue forever without repeating. Two numbers are in the golden ratio if their sum divided by the larger number equals the ratio of the larger number divided by the smaller number. Its relationship to investing was addressed in detail in the January/February issue of Luckbox. Mathematicians in India knew about the sequence as early as 200 B.C., and Leonardo of Pisa, who was later called Fibonacci, introduced it to the West in the 13th century. The golden ratio is related to the Fibonacci sequence of numbers, which begins with one and continues with the sum of the two preceding numbers. They’re in on the secret, and they see its almost magical qualities all around them. True believers choose to understand it as the simple pattern that underlies the complexity of the universe. They profess to perceive its presence even in the dimensions of the human face and body.
Some insist they see it reflected in the beguiling beauty of the Mona Lisa and embodied in the graceful architecture of structures as diverse as the Parthenon, the pyramids and the United Nations Secretariat Building. There’s little doubt that it appears in the principles of geometry, the pattern of a sunflower’s seeds and the angles formed by the branches of a tree.

Painters, sculptors, architects, composers and poets have embraced it for the structure it can provide. The golden ratio has been capturing the imagination of scholars for more than 2,000 years.Ĭountless mathematicians, physicists, botanists, chemists and psychologists have pondered its meaning.

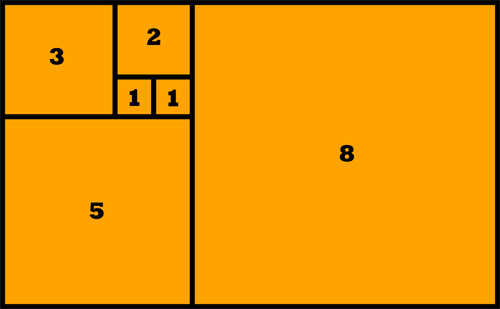
(In fact, the ratio is a number that begins 1.32472… and carries on forever).Leonardo da Vinci’s Vitruvian Man supposedly captured the perfect human dimensions, which some believe correspond to the golden ratio. It turned out that the ratio 1.325, which gives you the rectangle that creates the Harriss spiral has been written about – it is known as the “ plastic number” – but Harriss could find no previous drawings of the spiral. His first concern was that maybe someone else had had, in fact, drawn the spiral “One thing about mathematical discoveries and mathematical art is that even if the process is completely new there is no guarantee that someone else has not already explored it.” “It’s more difficult to make something mathematically satisfying that people haven’t seen before.” “It’s not hard to make something that no one has seen before,” he said. But he was particularly delighted because he arrived at the spiral using a very simple mathematical process. Harriss was overjoyed when he first saw the spiral because it was aesthetically appealing – one of his primary aims was to draw branching spirals like you might find in Islamic art or the work of Gustav Klimt.


 0 kommentar(er)
0 kommentar(er)
